Multiplikation och division i polär form
Repetition
- [math]\displaystyle{ cos (u+v) = cos u cos v - sin u sin v }[/math]
- [math]\displaystyle{ sin (u+v) = sin u cos v + cos u sin v }[/math]
- [math]\displaystyle{ cos (u-v) = cos u cos v + sin u sin v }[/math]
- [math]\displaystyle{ sin (u-v) = sin u cos v - cos u sin v }[/math]
Multiplikation
Vi kommer använda:
- [math]\displaystyle{ cos (u+v) = cos u cos v - sin u sin v }[/math]
- [math]\displaystyle{ sin (u+v) = sin u cos v + cos u sin v }[/math]
Två komplexa tal
- [math]\displaystyle{ z = r (cos u + i sin u) }[/math]
- [math]\displaystyle{ w = s (cos v + i sin v) }[/math]
Division med komplexa tal på polär form
Vid division av två komplexa tal dividerar men längderna på vektorerna:
- [math]\displaystyle{ | \frac{z}{w} | = \frac{|z|}{|w|} }[/math]
I analogi med multiplikationen så ska man subtrahera argumenten vid division av komplexa tal:
[math]\displaystyle{ arg \frac{z}{w} = arg z - arg w }[/math]
NP-uppgift

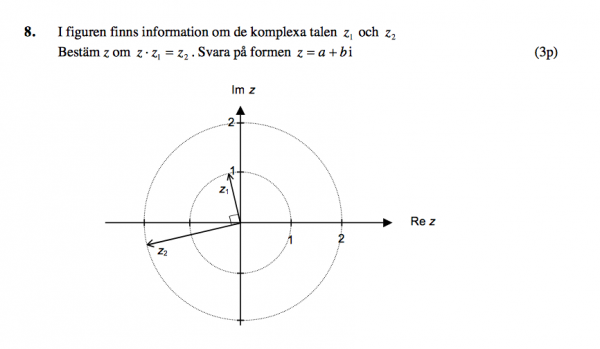
Uppgiften från Provbanken.