Tillämpningar på derivata: Skillnad mellan sidversioner
Hakan (diskussion | bidrag) |
Hakan (diskussion | bidrag) |
||
| Rad 31: | Rad 31: | ||
The simplest RC circuit is a capacitor and a resistor in parallel. When a circuit consists of only a charged capacitor and a resistor, the capacitor will discharge its stored energy through the resistor. The voltage across the capacitor, which is time dependent, can be found by using Kirchhoff's current law, where the current charging the capacitor must equal the current through the resistor. This results in the linear differential equation | The simplest RC circuit is a capacitor and a resistor in parallel. When a circuit consists of only a charged capacitor and a resistor, the capacitor will discharge its stored energy through the resistor. The voltage across the capacitor, which is time dependent, can be found by using Kirchhoff's current law, where the current charging the capacitor must equal the current through the resistor. This results in the linear differential equation | ||
:<math> | : <math> C\frac{dV}{dt} + \frac{V}{R}=0 </math>. | ||
C\frac{dV}{dt} + \frac{V}{R}=0 | |||
</math>. | |||
where C= capacitance of capacitor. | where C= capacitance of capacitor. | ||
Solving this equation for ''V'' yields the formula for exponential decay: | Solving this equation for ''V'' yields the formula for exponential decay: | ||
:<math> | |||
V(t)=V_0 e^{-\frac{t}{RC}} \ , | :<math> V(t)=V_0 e^{-\frac{t}{RC}} \ ,</math> | ||
</math> | |||
where ''V<sub>0</sub>'' is the capacitor voltage at time ''t = 0.'' | where ''V<sub>0</sub>'' is the capacitor voltage at time ''t = 0.'' | ||
| Rad 45: | Rad 43: | ||
The time required for the voltage to fall to <math>\frac{V_0}{e}</math> is called the RC time constant and is given by | The time required for the voltage to fall to <math>\frac{V_0}{e}</math> is called the RC time constant and is given by | ||
:<math> \tau = RC \ . </math> | : <math> \tau = RC \ . </math> | ||
==== Uppgift ==== | ==== Uppgift ==== | ||
Rita trafen för <math V(t) </math> och bestäm tidskonstanten <math> \Tau </math>. | Rita trafen för <math> V(t) </math> och bestäm tidskonstanten <math> \Tau </math>. | ||
Sätt in lämpliga värden R och Ci din funktion, gärna med glidare. | Sätt in lämpliga värden R och Ci din funktion, gärna med glidare. | ||
Versionen från 30 januari 2016 kl. 10.18
Digitala övningar
En snygg funktion
Undersök funktionen [math]\displaystyle{ f(x) = \frac{sin(x)}{x} }[/math].
- För vilket [math]\displaystyle{ x }[/math] har funktionen sitt största och minsta värde?
- Vad är gränsvärdet [math]\displaystyle{ \lim_{x \to \infty} f(x) }[/math] ?
- För vilka [math]\displaystyle{ x }[/math] är [math]\displaystyle{ f'(x) = 0 }[/math] ?
Talet e
Rita grafen för [math]\displaystyle{ f(x) = (1 + \frac{1}{x}^x }[/math] som du känner från avsnittet Beräkning_av_gränsvärden.
Skissa hur derivatan kan tänkas se ut genom att lägga in några punkter i GeoGebra.
Lägg in funktionen [math]\displaystyle{ g(x) = f'(x) }[/math] och se hur bra du lyckades med din skiss.
GeoGebra har även tagit fram derivatan som du ser och kan förundras över. Den följer inte våra enkla deriveringsregler.
RC-kretsen
Följande finns att läsa på Wikipedia: RC_cicuit:
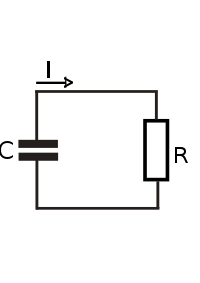
The simplest RC circuit is a capacitor and a resistor in parallel. When a circuit consists of only a charged capacitor and a resistor, the capacitor will discharge its stored energy through the resistor. The voltage across the capacitor, which is time dependent, can be found by using Kirchhoff's current law, where the current charging the capacitor must equal the current through the resistor. This results in the linear differential equation
- [math]\displaystyle{ C\frac{dV}{dt} + \frac{V}{R}=0 }[/math].
where C= capacitance of capacitor.
Solving this equation for V yields the formula for exponential decay:
- [math]\displaystyle{ V(t)=V_0 e^{-\frac{t}{RC}} \ , }[/math]
where V0 is the capacitor voltage at time t = 0.
The time required for the voltage to fall to [math]\displaystyle{ \frac{V_0}{e} }[/math] is called the RC time constant and is given by
- [math]\displaystyle{ \tau = RC \ . }[/math]
Uppgift
Rita trafen för [math]\displaystyle{ V(t) }[/math] och bestäm tidskonstanten [math]\displaystyle{ \Tau }[/math].
Sätt in lämpliga värden R och Ci din funktion, gärna med glidare.
